The Art & Science of Tuning
Piano tuning is not nearly as easy as it looks.
Guus van den Braak makes it look easy because he is a very experienced expert. He has tuned professionally thousands of pianos since 1970. Most people grossly underestimate how skillful and amazingly complex it is. Very few people can do the work. It requires very careful listening and intense concentration. Making extremely fine adjustments to string tension by turning very tightly fitted tuning-pins ever so minuscule is physically very strenuous, and extremely skillful work. It takes about 8 to 10 kg/force to turn a tuning-pin, and there are some 225 strings and tuning-pins. The tuner leans, bends and twists over the piano to reach the tuning-pins whilst making extremely fine turning adjustments to the pins and playing the keys at the same time. Just think about how his head, back, neck and shoulders would feel after standing bent and twisted in one spot, “arm wrestling” for about 1½ hours, and intensely listening to sound. Piano tuners well and truly earn their money. The tuner compares one pitch to another and adjusts the tension of strings so they sound pleasant when played in combinations. Pitches of nearly coincident frequencies produce beats or pulsations in the loudness of the tone. When comparing pitches, you listen for beats, and depending on the interval, you either eliminate them or adjust their speed.
But there is much more to the art of tuning a piano than meets the average eye, and ear. It is not something you learn and master in a few weeks or even a few years. Most people grossly underestimate how intense the training is and how difficult it is to become a highly skilled tuner/technician. To be successful, you would really want to do this, be very patient and determined. Just as one does not become a highly competent pianist in one year of training, neither does one become a competent all-round tuner/technician in that time frame. It takes many more years to further develop one’s skills and knowledge. Just as a person needs to invest many thousand of dollars in a good quality instrument and education to become a professional pianist, a similar investment in training and tools needs to be made to become a professional piano tuner and technician who is capable of not only tuning a piano properly, but who is also able to rebuild a piano and make the instrument sound, and mechanically perform at its optimum. One would need to be a good multi-skilled wood and metal worker, have excellent hearing, have fine motor skills, continue to study, practice every day, and be coached by a good tuner/ technician for many years.
A vibrating string subdivides itself into many simultaneously vibrating segments. Each segment produces an audible pitch of its own, called an overtone or partial. Piano tuners talk about partials, not overtones. When we play a chord with other strings, we want not only the fundamentals to match up but also the partials.
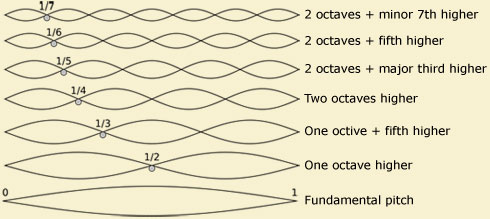
Strings do not vibrate according to theory. A harmonic is a theoretical frequency that is an exact multiple of the fundamental tone. String stiffness causes the vibrating segments to produce partials that are not true harmonics. In reality, string wire stiffness causes the partials of strings struck by piano hammers to be sharp of their theoretical harmonics. The sharpness, or distortion of partials from the true harmonic series, is called inharmonicity. Overtones are like partials, but are numbered differently. A first partial is a fundamental, and a second partial is the first overtone.
Tuning an octave and the rest of the piano to perfection in Equal Temperament is one thing, but to make matters worse, a piano has three strings to the note and the three strings need to sound as one string, without any beat (a perfect unison). If you have ever tried to tune a 12-string guitar to perfection, you will start to get the idea. So, if you see someone tuning a guitar and he is very lightly touching the strings to do so-called harmonic tuning, he is in fact tuning with partials (overtones) and not harmonics.
To make matters more complex, usually octaves are in reality not double the frequency as most people assume, but are stretched ever so slightly. Guus van den Braak knows how much to stretch octaves over the entire 88 keys. This octave stretch differs from one piano to the next. Very small pianos should have very little to no stretch and the octaves should be “clean”, whilst a 9-foot concert grand should have more widely stretched octaves. If a 9-foot concert grand was tuned with little to no stretch, it would not sound as good as it could. Likewise, if a very small piano was tuned with more widely stretched octaves, it would also not sound as good as it could. This has to do with inharmonicity.
Often, tuners have to deal with neglected pianos, which have tonally deteriorated to such extent that many strings have a false beat of their own (a vibrato-like sound). Trying to make these three strings to sound like one (a perfect unison) is as good as impossible and tuning has become a nightmare. If we are lucky we may be able to tune the false beating strings “out of phase”, but this is extremely difficult and simply not possible if the three false beating strings beat at a different speed. If possible, it shortens the sustain of that note, and the so-called “perfect” unison will not last long. Guus van den Braak “does the impossible, but does not perform miracles.”
Tuning history
Pythagoras developed a system called the Circle of Fifths. For each following note, he multiplied the frequency by three and divided it by two. This produced a nice overtone effect, but there was a shortfall in the sequence because it did not quite reach a perfect octave (twice the frequency of the first note – e.g. A3=220 hertz to A4=440hertz).
Sometime later, the “Just Temperament” was developed where all notes in an octave were simply whole number ratios of the fundamental note, such as 6/5, 5/4 and 3/2. Violins and cellos have no frets and are naturally tuned and played that way.
However, if we were to tune a piano in the historical “Just Temperament” in the key of A, the ratios would not work in the key of C and the tuning would sound wrong and unpleasant. You would need a different piano, tuned in the key of C, to be able to play a different piece of music in the key of C.
The “Equal Temperament” was developed in the 16th Century in which each semitone step is an equal ratio. The only problem is that the “Equal Temperament” ratio is an irrational number of the 12th root of two. This number (1.0594631) cannot be expressed as a simple whole number fraction, and you will never quite get a perfect tuning with the partials (overtones) in tune. Putting it a different way, “Equal Temperament” is a compromise, which is equally out of tune for each note in an octave. However, our amazing brain also makes compromises and hears (imagines) the correct pitch.

